Radical Equations
The Power Rule
If x, y, and n are real numbers and 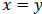 , then , then 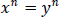 . .
*Be Careful!!! Consider x=3.
Equations Containing One Radical
Example 1: Solve: 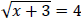
Check:
Example 2: The distance d (in feet) that an object will
fall in t seconds is given by the formula  . .
To find the height of a bridge a man drops a stone from the bridge into water
below. If it took 3 seconds
to hit the water, how far above the river is the bridge?
Check:
*Note: Always isolate the radical on one side before
raising both sides of the equation to a power.
Example 3: Solve: 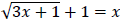
Check:
Example 4: Solve: 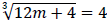 . .
Check:
Equations Containing Two Radicals
Example 5: Solve: 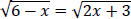 . .
Check:
When more than one radical appears in an equation, it is often necessary to
apply the power rule more
than once.
Example 6: Solve: 
Check:
Solving Formulas Containing Radicals
Recall: To solve a formula for a variable means to isolate that variable on one
side of the equation.
Example 7: Some office equipment that is now worth V dollars originally cost C
dollars 3 years ago. The
rate r at which it has depreciated is given by
 . Solve the formula for C. . Solve the formula for C.
|