Radicals and Rational Exponents
Definition of the Principal Square
Root• If a is a nonnegative real number,
the
nonnegative number b such that b2 = a,
denoted by 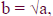 is the principal square is the principal square
root of a. |
| Square Roots of Perfect Squares
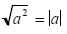 |
The Product Rule for Square Roots
• If a and b represent nonnegative real
number, then

• The square root of a product is the product
of the square roots. |
Text Example
• Simplify 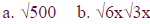
Solution:
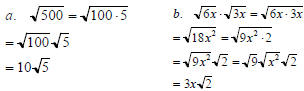 |
| The Quotient Rule for Square Roots
• If a and b represent nonnegative real
numbers and b does not equal 0, then
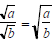 and and
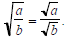
• The square root of the quotient is the
quotient of the square roots. |
| Text Example •
Simplify: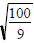
Solution: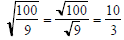 |
Example
• Perform the indicated operation:
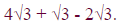
Solution: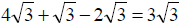 |
Example
• Perform the indicated operation:
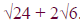
Solution:
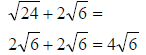
|
Definition of the Principal nth Root
of a Real Number
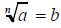 means that means that
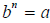
• If n, the index, is even, then a is
nonnegative (a≥ 0) and b is also
nonnegative (b ≥ 0) . If n is odd, a and b can
be any real numbers. |
Finding the nth Roots of Perfect
nth PowersIf n is odd,
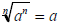
If n is even an 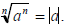 |
The Product and Quotient Rules
for nth Roots
• For all real numbers, where the indicated
roots represent real numbers,
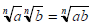 and and
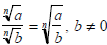 |
| Definition of Rational Exponents
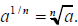
Furthermore,
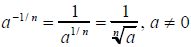
|
| Example • Simplify
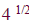
Solution:
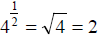
|
| Definition of Rational Exponents

• The exponent m/n consists of two parts: the
denominator n is the root and the numerator
m is the exponent. Furthermore,
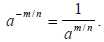
|
Radicals and
Rational Exponents |
|